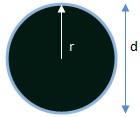
r: radius
d: diameter
A: Area

The area of a circle is given by:

A = Π ∙ r² or A = Π ∙ (d/2)²

Π defines the ratio of any circle's circumference to its diameter and is approximately equal to 3.141593, however the value 3.14 is often used.

Example 1: The diameter of a circle is 16 inches. What is the lateral area?

Solution: A = Pi * r²

Radius = d/2 = 16/2 = 8

A = 3.14 * 8²   

A = 3.14 * (64)  

A = 200.96 cm²

Example 2: From a circular sheet of radius 6 cm, a circle of radius 3 cm is removed. Find the area of the remaining sheet.

Solution: Here, outer radius, R = 6 cm

Inner Radius, r = 3 cm

Area of remaining sheet = Outer area - Inner area

                                             = Pi * (R² - r²)

                                             = 3.14 ( 6²  - 3²)

                                             = 3.14 (36 - 9)

                                             = 3.14 x 27

                                             = 84.78 cm²

Hence the area of remaining sheet is 28.26 cm²

Example 3: Find the radius of the circle when its area is known to be 56.52 square centimeters.

Solution: We know that the area of the circle is given by: A = Pi * r²

Isolating r we will get: r² = A / Pi or r = √(A/Pi)

Thus:

r = √(56.52/3.14) = √(18) centimeters

Example 4: Vandana uses a lawn sprinkler to water the circular region of the lawn having 30 ft in diameter. Calculate the area watered on the lawn.

Solution:

Step 1: The shape of watered region is circular. Formula for area A = Pi * r² where r is the radius of the circle.
Step 2: The diameter of the circular region is 30 ft i.e. r = 30/2 = 15ft
A = Pi * r²

= 3.14 * 15²

=706.5 ft²

Example 5: Determine the area of a circle whose radius is 5 meter.
A = Π ∙ r² = 3.14 ∙ 5² = 78.5 m²

Example 6: Determine the area of a circle whose diameter is 10 meter.
A = Π ∙ (d/2)² = 3.14 ∙ (10/2)² = 78.5 m²
Note that radius = diameter / 2

Online Area Calculator